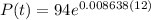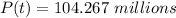Question

A country's population in 1993 was 94 million in 1999 it was 99 million estimate population in 2005 using the exponential growth formula

Answer 1

104 million

Explanation:

We are given that a country's population in 1993 was 94 million and in 1999, it was 99 million.

We are to estimate its population in 2005.

Let P(t) denote the population at t years after 1993, then:

P(0) = 94 million

P(6) = 99 million

`P(t) = P(0) e^((k t) )`

So
`P(6) = P(0) e^((6 k) )`

`99 = 94 e^((6 k) )`

`e^((6 k)) = (99)/(94)`

`6k=ln((99)/(94) )`

`k=ln(((99)/(94))/(6)  )`

`k=0.0086375`

Now since we have found the value of
`k`, we can estimate the population in 2005:

t = 2005 - 1993 = 12

P (12) =
`P(0) e^(( 12 k)) = 94 e^(( 12 (0.0086375) ) )` = 104.266 million

Answer 2

`P(t) = 104.267\ millions`

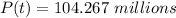
Explanation:

The exponential growth formula is

`P(t) = Ae^(kt)`

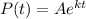
Where

A is the main coefficient and represents the initial population.

e is the base

k is the growth rate

t is time in years.

Let's call t = 0 to the initial year 1993.

At t = 0 the population was 94 millions

Therefore we know that:

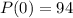`P(0) = 94` millions

After t = 6 years, in 1996, the population was 99 millions.

Then
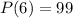`P(6) = 99` million.

Now we use this data to find the variables a, and k.

`P(0) = 94 =Ae ^(k(0))\\\\94 = A(e ^ 0)\\\\A = 94`.

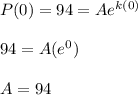Then:

`P(6) = 94e^(k(6))\\\\99 = 94e ^(6k)\\\\(99)/(94) = e^(6k)\\\\ln((99)/(94)) = 6k\\\\k = (ln((99)/(94)))/(6)\\\\k =0.008638`

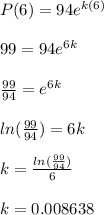
Finally the function is:

`P(t) = 94e^(0.008638t)`

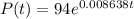
In the year 2005:

`t = 2005-1993\\\\t= 12\ years`

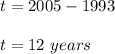
So the population after t=12 years is:

`P(t) = 94e^(0.008638(12))`

`P(t) = 104.267\ millions`