Question

Which of the following polynomials has solutions that are not real numbers?

A)x^2-6x+3
B)x^2+4x+3
C)-x^2-9x-10
D)x^2+2x+3

Answer 1

D)
`x^(2) + 2x + 3`.

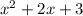Explanation:

All four polynomials are quadratic, meaning that the highest power of the unknown
`x` in the equation is two.

The sign of the quadratic discriminant,
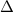`\Delta`, is a way to tell if a quadratic polynomial comes with non-real solutions.

There are three cases:

• 
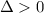  `\Delta > 0`. The quadratic discriminant is positive. There are two real solutions and no non-real solution. The two solutions are different from each other.
• 
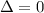  `\Delta = 0`. The quadratic discriminant is zero. There is one real solution and no non-real solution.
• 
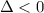  `\Delta <0`. The quadratic discriminant is negative. There is no real solution and two non-real solutions.

How to find the quadratic discriminant?

If the equation is in this form:

`a \; x^(2) + b\;x + c = 0`,

where a, b, and c are real numbers (a.k.a. "constants.")

Quadratic discriminant:

`\Delta = {b^(2) - 4\;a\cdot c}`.

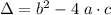Polynomial in A:

`x^(2) - 6x + 3 = 0`.

• 
  `a = 1`.
• 
  `b = -6`.
• 
  `c = 3`.

`\Delta = b^(2) - 4 \;a\cdot c = (-6)^(2) - 4* 1* 3 = 36 - 12 = 24`.

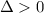`\Delta > 0`. There will be no non-real solutions and two distinct real solutions.

Try the steps above for the polynomial in B, C, and D.

• B):
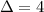  `\Delta = 4`. Two distinct real solutions. No non-real solution.
• C):
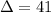  `\Delta= 41`. Two distinct real solutions. No non-real solution.
• D):
  `\Delta = -8`. No real solution. Two distinct non-real solutions.