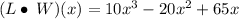Question

The length of a rectangle is represented by the function L(x) = 5x. The width of that same rectangle is represented by the function W(x) = 2x2 − 4x + 13. Which of the following shows the area of the rectangle in terms of x? (L ⋅ W)(x) = 10x3 − 4x + 13 (L ⋅ W)(x) = 10x3 − 20x2 + 65x (L + W)(x) = 2x2 + 1x + 13 (L + W)(x) = 2x2 − 9x + 13

Answer 1

B/Second Answer: (L • W)(x) = 10x^3 − 20x^2 + 65x

Answer 2

`(L \bullet \: W)(x)= 10 {x}^(3) - 20 {x}^(2) + 65x`

Step-by-step explanation

The length of a rectangle is represented by the function:

`L(x) = 5x`

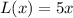
and the width is represented by

`W(x)= 2 {x}^(2) - 4x + 13`

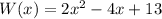
The area of a rectangle is calculated using the formula:

`Area = LW`

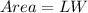
In terms x, we have

`Area = (L \bullet \: W)(x)= L(x) * W(x)`

We plug in the functions representing the length and width to obtain;

`(L \bullet \: W)(x)= 5x(2 {x}^(2) - 4x + 13)`

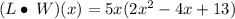
We expand the parenthesis to obtain;

`(L \bullet \: W)(x)= 10 {x}^(3) - 20 {x}^(2) + 65x`