Question

A simple pendulum consists of a brass sphere of mass m = 1 kg suspended on a string of length L ≈ 1 meter. The pendulum oscillates with amplitude A = 1 cm and period T = 2 seconds. Which of the following alterations will change the period of the pendulum’s oscillations to T ′ = 1 second? 1. Using a lighter brass sphere of mass m ′ ≈ 0.25 kg. 2. Using a shorter string of length L ′ ≈ 0.25 meters. 3. Using a longer string of length L ′ ≈ 2 meters. 4. Using a longer string of length L ′ ≈ 4 meters. 5. Using a shorter string of length L ′ ≈ 0.5 meters. 6. Increasing the oscillation amplitude to A ′ = 4 cm. 7. Using a heavier brass sphere of mass m ′ ≈ 4 kg. 8. Reducing the oscillation amplitude to A ′ = 0.25 cm.

Answer 1

2. Using a shorter string of length L ′ ≈ 0.25 meters

Step-by-step explanation:

The period of a pendulum is given by

`T = 2\pi \sqrt{(l)/(g) }`

where

L is the length of the pendulum

g is the acceleration due to gravity

Looking at the formula, you can rule out every answer that includes mass or amplitude because the equation only depends on the string length.

Since gravity is a constant, you can ignore it in this case. Since the period is now proportional to the square root of the length take the square root of each possible length until you find that the square root of 0.25 is 0.5.

Since 2 seconds multiplied by 0.5 is 1 second, the length of the string should be 0.25 meters.

Therefore, the only alteration that will change the period of the pendulum’s oscillations to T ′ = 1 second is using a shorter string of length L ′ ≈ 0.25 meters.

Answer 2

2. Using a shorter string of length L ′ ≈ 0.25 meters

5. Using a shorter string of length L ′ ≈ 0.5 meters

Step-by-step explanation:

The period of a pendulum is given by

`T=2\pi \sqrt{(L)/(g)}`

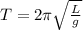
where

L is the length of the pendulum

g is the acceleration due to gravity

We see from the formula that the period of the pendulum depends only on its length, not on its mass or its amplitude of ocillation. Therefore, the only alterations that can change the period of the pendulum are the ones where its length is changed.

Moreover, we notice that the period is proportional to the square of the length: this means that in order to decrease the period of the pendulum (the problem asks us which alterations will reduce the period of the pendulum from 2 s to 1 s), the length of the pendulum should also be reduced.

Therefore, the only alterations that will reduce the period of the pendulum are:

2. Using a shorter string of length L ′ ≈ 0.25 meters

5. Using a shorter string of length L ′ ≈ 0.5 meters