Answer: The correct option is (A) 58 cm.
Step-by-step explanation: We are given to find the length of MN from the figure shown.
From the figure, we note that
in triangle ABC, M is the midpoint of AB and N is the midpoint of BC.
Also, AM = 88 am, BN = 75 cm and AC = 116 cm.
The Midpoint Theorem : It states that the segment joining two sides of a triangle at the midpoints of those sides is parallel to the third side and is half the length of the third side.
Therefore, applying Midpoint theorem in triangle ABC, we find that
MN is parallel to AC and is half the length of AC.
That is,
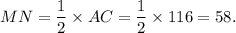
Thus, the length of MN is 58 cm.
Option (A) is CORRECT.