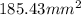Question

The radius of the semicircle in the following composite figure is 8.5 millimeters. What is the total area of the composite figure rounded to the nearest hundredth?

Answer 1

The area is : 226.865

Rouded off : 230

Answer 2

`185.43 mm^2`

Explanation:

Radius of semicircle = 8.5 mm

Area of semicircle =
`(\pi r^2)/(2)`

=
`(3.14 (8.5)^2)/(2)`

=
`113.4325 mm^2`

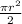
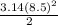
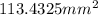
Area of triangle =
`(1)/(2) * Base * Height`

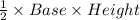
Area of triangle in figure=
`(1)/(2) * 12 * 12`

=
`72 mm^2`

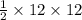
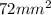
So, Area of the figure = Area of semicircle +Area of triangle

=
`113.4325 mm^2+72 mm^2`

=
`185.43 mm^2`

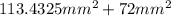
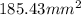
Thus Option B is correct.

Hence the total area of the composite figure rounded to the nearest hundredth is
`185.43 mm^2`