Answer:

Explanation:
Given that , Paul needs 27 plants to fill his garden and has $99 to spend. Lilies cost $5 each and tulips cost $2 each.
- Tulips are represented by t .
- Lilies are represented by l .
Now we need to find which set of linear equations represent the above situation. So ,
Sum of number of lilies and tulips is 27 .
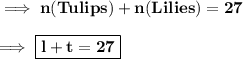
Now , cost of each lily is $5 and tulip is $2 . And he has $99 in total . So ,
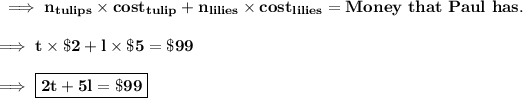
Hence we can see that our ans matches with option (a) which is ,
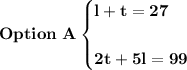
Hence option (A) is correct .