Question

Which equation correctly shows how to determine the distance between the points (9, –2) and (6, 3) on a coordinate grid?

Answer 1

`d = \sqrt{ {(6 - 9)}^(2) + {(3 - ( - 2))}^(2) }`

EXPLANATION

To find the distance between any two points

`(x_1,y_1)`

and

`(x_2,y_2)`

We use the distance formula;

`d = √((x_2-x_1)^2 +(y_2-y_1)^2)`

We let

`(x_1,y_1) = (9, - 2)`

and

`(x_2,y_2) = (6, 3)`

We substitute the coordinates into the formula to get;

`d = \sqrt{ {(6 - 9)}^(2) + {(3 - ( - 2))}^(2) }`

The third choice is correct.

Answer 2

Answer:
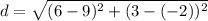`d=√((6-9)^2+(3-(-2))^2)` (Third option)

Explanation:

The formula for calculate the distance (d) between two points is:

`d=√((x_2-x_1)^2+(y_2-y_1)^2)`

Given the points (9,-12) and (6,3), you can identify:

`x_2=6\\x_1=9\\y_2=3\\y_1=-2`

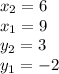
Substitute these coordinates into the formula
`d=√((x_2-x_1)^2+(y_2-y_1)^2)` to find the equation that correctly shows how to determine the distance between the given points (9,-12) and (6,3).

Then:

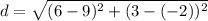`d=√((6-9)^2+(3-(-2))^2)` (Third option)