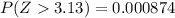Answer:
Explanation:
A set of normally distributed data has a mean of 3.2 and a standard deviation of 0.7. Find the probability of randomly selecting 30 values and obtaining an average greater than 3.6.
We can denote the population mean with the symbol

According to the information given, the data have a population mean:
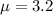 .
.
The standard deviation of the data is:
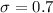 .
.
Then, from the data, a sample of size
 is taken.
is taken.
We want to obtain the probability that the sample mean is greater than 3.6
If we call
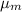 to the sample mean then, we seek to find:
to the sample mean then, we seek to find:
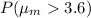
To find this probability we find the Z statistic.
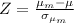
Where:
Where
 is the standard deviation of the sample
is the standard deviation of the sample
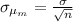
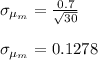
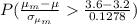
Then:
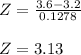
The probability sought is:
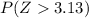
When looking in the standard normal probability tables for right tail we obtain: