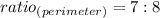Answer: first option.
Explanation:
The ratio of the area of the triangles can be calculated as following:

Where
 is the lenght of the given side of the smaller triangle and
is the lenght of the given side of the smaller triangle and
 is the lenght of the given side of the larger triangle.
is the lenght of the given side of the larger triangle.
Therefore:

It can be written as following:

The ratio of the perimeter is:
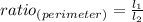
Where
 is the lenght of the given side of the smaller triangle and
is the lenght of the given side of the smaller triangle and
 is the lenght of the given side of the larger triangle.
is the lenght of the given side of the larger triangle.
Therefore:
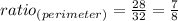
It can be written as following: