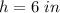Question

The height and diameter of a cylindrical cup are the same length. The volume of the cup is 54pi in3 what is the radius and the height of the cup

Answer 1

The radius of the cup is
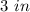`3\ in` and

the height of the cup is
`6\ in`

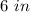
Explanation:

we know that

The volume of the cylinder is equal to

`V=\pi r^(2)h`

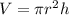
Let

x-----> the height and the diameter of the cylinder

we have

`V=54\pi\ in^(3)`

`h=x\ in`

`D=x\ in`

`r=(x/2)\ in`

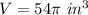
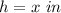
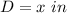
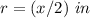
substitute the values

`54\pi=\pi (x/2)^(2)(x)`

simplify

`54=(x/2)^(2)(x)`

`54=(x^(3))/(4) \\ \\x^(3)=216\\ \\x=6\ in`

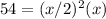
therefore

The radius of the cup is

`r=(6/2)=3\ in`

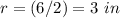
The height of the cup is

`h=6\ in`