Question

Which number line shows the solution set for |d| > 3?

Answer 1

Last option

`d>3` or
`d<-3`

Explanation:

The absolute value is a function that transforms any value x into a positive number.

Therefore, for the function
`f(x) = |x|` x> 0 for all real numbers.

Then the inequation:

`|d|> 3` has two cases

`(d)` if
`d>0` (i)

`-(d)` if
`d< 0` (ii)

We solve the case (i)

`d> 3`

We solve the case (ii)

`-d>3\\d < -3`

Then the solution is:

`d>3` or
`d<-3`

Answer 2

Last choice is the correct graph.

Explanation:

We have been given inequality
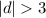`|d|>3`. Now we need to find out which of the given number lines shows the correct solution set for
`|d|>3`.

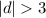We know that
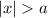`|x|>a` can be broken into :

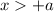`x>+a` or
`x<-a`

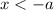
Same way we can break
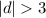`|d|>3` into two parts as:

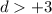`d>+3` or
`d<-3`

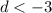
Since it has only < symbol but not equal so we make an open circle at both +3 and -3.

Hence last choice is the correct graph.