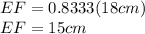Question

Triangle ABC is similar to triangle DEF. The length of

AC is 12 cm. The length of
BC is 18 cm. The length of
DF is 10 cm.

What is the length of
EF?

Answer 1

The length of EF = 15 cm.

Explanation:

We are given that the two triangles, ABC and DEF, are similar to each other,

Given that the length of AC = 12 cm, BC = 18 cm and DF = 10 cm, we are to find the length of EF.

For this, we can simply use the ratio method.

`\frac { EF } { BC } = \frac { DF } { AC }`

`\frac { EF } { 18 } = \frac { 10 } { 12 }`

`E F = \frac { 10 } { 12 } * 18`

EF = 15 cm

Answer 2

Answer:
`EF=15cm`

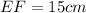
Explanation:

You know that the triangle ABC and the triangle DEF are similar.

Therefore, if the lenght of AC is 12 centimeters and the lenght of DF is 10 centimeters, then you can find the ratio as following:

`ratio=(DF)/(AC)\\\\ratio=(10cm)/(12cm)\\\\ratio=0.8333`

Then, the calculte the length of EF, you must multiply the lenght BC of the triangle ABC by the ratio obtained above.

Therefore, the lenght EF is the following:

`EF=0.8333(18cm)\\EF=15cm`