Question

Given a circle with measures of (C, d, and r) and a circle with measures of (C', d', and r'), what is d if C C' = 12 and d' = 0.50?

Answer 1

I think the D equals one (1) based on the info i was given

Explanation:

Answer 2

d = 6 units

Explanation:

Circumference of the circle is given by:

`C = \pi d`

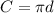
where, d is the diameter of the circle.

As per the statement:

Given a circle 1 with measures of (C, d, and r) and a circle 2 with measures of (C', d', and r').

Circumference of a circle(C) 1 with diameter(d) is given by:

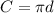`C = \pi d` .....[1]

Also, the circumference of a circle (C') 2 with diameter(d') is given as:

`C' = \pi d'`

Divide equation [1] and [2] we have;

`(C)/(C') = (\pi d)/(\pi d')`

⇒
`(C)/(C') = (d)/(d')`

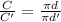
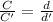
Substitute the given values
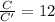`(C)/(C')=12` and d' = 0.50 then;

`12 = (d)/(0.50)`

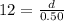
Multiply both sides by 0.50 we have;

6 = d

or

d = 6 units

Therefore, the value of d is, 6 units