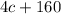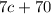Question

Hailey and Kacie are selling candles to raise money. • Hailey is selling candles for $4 each and has already raised $160. • Kacie is selling candles for $7 each and has already raised $70. Which equation can be used to find c, the number of candles each girl needs to sell so that the total amount raised is the same for both Hailey and Kacie?

Answer 1

160+4h=70+7k

Explanation:

h(160))

k(70)

4h=7k

Answer 2

Answer: Our required equations would be

`4c+160`

`7c+70`

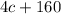
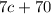
Explanation:

Let the number of candles be 'c'.

In case of Hailey:

Cost of each candle = $4

Amount already raised = $160

So, our equation becomes

`4c+160`

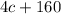
In case of Kacie:

Cost of each candle = $7

Amount already raised = $70

So, our equation becomes,

`7c+70`

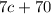
According to question, total amount raised is same for both the cases ,

so, it becomes,

`4c+160=7c+70\\\\160-70=7c-4c\\\\90=3c\\\\c=(90)/(3)\\\\c=30`

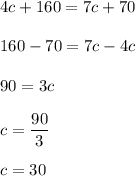
Hence, our required equations would be

`4c+160`

`7c+70`