Question

In a city school, 60% of students have blue eyes, 55% have dark hair, and 35% have blue eyes and dark hair. What is the probability (rounded to the nearest whole percent) that a randomly selected student will have dark hair, given that the student has blue eyes?

Hint:
P(A|B)=P(A∩B) / P(B)

64%

58%

80%

20%

Answer 1

58%

Explanation:

This is a problem of conditional probability.

Let A represent the event that student has dark hair.

So P(A) = 55% = 0.55

Let B represents the event that student has blue eyes.

So, P(B) = 60% = 0.60

Probability that student has blue eyes and dark hairs = P(A and B) = 35% = 0.35

We are to find the probability that a randomly selected student will have dark hair, given that the student has blue eyes. Using the given formula and values, we get:

`P(A|B)=(P(A \cap B))/(P(B))\\\\ P(A|B)=(0.35)/(0.60)\\\\ P(A|B)=0.58`

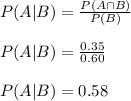
Therefore, there is 0.58 or 58% probability that the student will have dark hairs, given that the student has blue eyes.