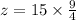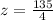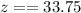Question

Z varies inversely with x and directly with y. When x=6 and y=2 z=5 what is the value of a when x=4 and y=9

Answer 1

15·9

z = --------- = 135/4

4

Explanation:

The general formula here is as follows:

ky

z = ---------

x

We must find the value of the constant of proportionality. To do this, subst. 6 for x, 2 for y and 5 for z and compute k:

k·2

5 = ---------

6

Multiplying both sides by 6 results in 30 = 2k.

Thus, k = 30/2, or k = 15.

Our formula becomes:

15y

z = ---------

x

if x = 4 and y = 9, we get:

15·9

z = --------- = 135/4

4

Answer 2

`z=33.75`

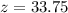
Explanation:

We are given that z varies inversely with x and directly with y when x=6 and y=2 then z=5

We have to find the value of z when x=4 and y=9

According to question

`z\propto(1)/(x)`

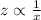
and
`z\proptoy`

Therefore, we have
`z\propto(y)/(x)`

`z=k(y)/(x)`

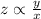
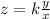
k=proportionality constant

Substitute x=6 , y=2 and z=5 then we get

`5=k* (2)/(6)`

`5=(k)/(3)`

`k=5*3`

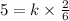
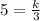
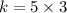
By division poperty of equality

k=15

Now, substitute the value x=4,y=9 and k=15 then the value of z

`z=15* (9)/(4)`

`z=(135)/(4)`

`z==33.75`