Question

Identify the graph of 2x^2+2y^=9 for theta=30º and write and equation of the translated or rotated graph in general form.

Answer 1

The answer is D

Good luck on the Ed-genuity test

Answer 2

The answer is circle; (x')² + (y')² - 4 = 0

Explanation:

* At first lets talk about the general form of the conic equation

- Ax² + Bxy + Cy² + Dx + Ey + F = 0

∵ B² - 4AC < 0 , if a conic exists, it will be either a circle or an ellipse.

∵ B² - 4AC = 0 , if a conic exists, it will be a parabola.

∵ B² - 4AC > 0 , if a conic exists, it will be a hyperbola.

* Now we will study our equation:

* 2x² + 2y² = 8

∵ A = 2 , B = 0 , C = 2

∴ B² - 4AC = (0) - 4(2)(2) = -16 < 0

∵ B² - 4AC < 0

∴ it will be either a circle or an ellipse

* Lets use this note to chose the correct figure

- If A and C are equal and nonzero and have the same sign,

then the graph is a circle.

- If A and C are nonzero, have the same sign, and are not equal

to each other, then the graph is an ellipse.

∵ A = 2 and C = 2

∴ The graph is a circle.

∵ D and E = 0

∴ The center of the circle is the origin (0 , 0)

∵ Ф = 30°

∴ The point (x , y) will be (x' , y')

- Where x = x'cosФ - y' sinФ and y = x'sinФ + y'cosФ

∴ x = x'cos(30°) - y'sin(30°)

∴ y = x'sin(30°) + y'cos(30°)

∴ x = (√3/2)x' - (1/2)y' and y = (1/2)x' + (√3/2)y'

∴
`x=(√(3)x'-y')/(2)`

∴
`y=(x'+√(3)y')/(2)`

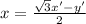
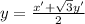
* Lets substitute x and y in the first equation

∴
`2((√(3)x'-y')/(2))^(2)+2((x'+√(3)y')/(2))^(2)=8`

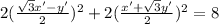
* Use the foil method

∴
`2((3x'^(2)-2√(3)x'y'+y'^(2))/(4))+2((x'^(2)+2√(3)x'y'+3y'^(2))/(4))=8`

* Open the brackets

∴
`(3x'^(2)-2√(3)x'y'+y'^(2)+x'^(2)+2√(3)x'y'+3y'^(2))/(2)=8`

* Collect the like terms

∴
`(4x'^(2)+4y'^(2))/(2)=8`

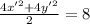
* Simplify the fraction

∴ 2(x')² + 2(y')²= 8

* Divide each side by 2

∴ (x')² + (y')² = 4

∴ The equation of the circle is (x')² + (y')² = 4

* The general equation of the circle is (x')² + (y')² - 4 = 0

after rotation 30° about the origin

* Look to the graph

- The blue circle for the equation 2x² + 2y² = 8

- The blue circle for equation (x')² + (y')² - 4 = 0

* That is because the two circles have same centers and radii

- The green line is x' and the purple line is y'