Question

Write and equation of the translated or rotated graph in general form (picture below)

Answer 1

Option b

Explanation:

The equation
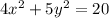`4x ^ 2 + 5y ^ 2 = 20` has center in (0,0).

But the transformation
`T(5, -6)` shifts the center of the equation to the point (5, -6).

Therefore, when applying
`T(5, -6)` we will have the following equation translated.

`4(x-5) ^ 2 + 5(y - (-6)) ^ 2 = 20`.

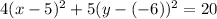Simplifying we have:

`4(x-5) ^ 2 + 5(y + 6) ^ 2 = 20`

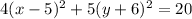
Now we expand
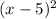`(x-5) ^ 2` and
`(y + 6) ^ 2`

`4(x ^ 2 -10x +25) + 5(y ^ 2 + 12y +36) = 20\\\\4x ^ 2 -40x + 100 + 5y ^ 2 + 60y + 180 = 20\\\\4x ^ 2 + 5y ^ 2 -40x + 60y +260 = 0`

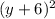
The equation of a circle has the form

`h(x-a) ^ 2 + q(y-b) ^ 2 = r`

For h = 1 and q = 1.

If
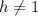`h \\eq 1` and
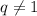`q\\eq 1` then the graph becomes an ellipse.

In this problem h = 4 and q = 5 therefore the figure is an ellipse