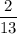Question

A bag contains 6 red balls, 4 green balls, and 3 blue balls. If we choose a ball, then another ball without putting the first one back in the bag, what is the probability that the first ball will be green and the second will be red?

Answer 1

First ball will be green- 4/13
Second ball will be red-6/12 or 1/2

First ball will be green AND second ball will be red- 4/13x1/2=4/26 or 2/13

Answer 2

Answer:
`(2)/(13)`

Explanation:

Given : A bag contains 6 red balls, 4 green balls, and 3 blue balls.

Total balls = 6+4+3=13

Probability of drawing first ball as green :

`P(G)=\frac{\text{Number of green balls}}{\text{Total balls}}\\\\=(4)/(13)`

If the first ball will be green, then the total balls left in bag = 13-1=12 and number of red balls remains the same.

Now, The conditional probability of drawing a red ball given that first ball was green :-

`P(R|G)=\frac{\text{Number of red balls}}{\text{Total balls left}}\\\\=(6)/(12)=(1)/(2)`

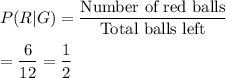
Now, the probability that the first ball will be green and the second will be red will be :-

`P(G\cap R)=P(G|R)* P(G)\\\\=(4)/(13)*(1)/(2)=(2)/(13)`

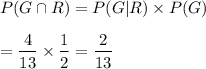
Hence, the required probability =
`(2)/(13)`