Answer: 15,625 ft³
Explanation:
You need to use the formula for calculate the volume of a cylinder:
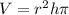
Where r is the radius and h is the height.
You know the radius and the volume of the old tank, therefore you can find the height as following:
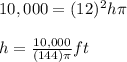
You know that the radius of the new tank is 15 feet and it has the same height as the old tank. Therefore, you can substitute h into the first equation for calculate the volume of a cylinder, to find the maximum number of cubic feet of water that the new storage tank will hold:
