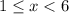Question

What is the solution to the compound inequality 3x − 8 ≥ −5 and 2x − 7 < 5?

x ≤ 1 and x > 6
1 ≤ x < 6
x > 1 and x > 6
1 < x < 6

Answer 1

1<x<6 or its x>1 and x>6

Answer 2

The solution to the compound inequality is given by:

`1\leq x<6`

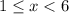
Explanation:

The compound inequality is given by:

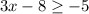`3x-8\geq -5` and

`2x-7<5`

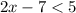
• On solving the first inequality i.e.

`3x-8\geq -5`

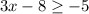
on adding both side of the inequality by 8 we get:

`3x\geq -5+8\\\\i.e.\\\\3x\geq 3`

Now on dividing both side of the inequality by 3 we get:

`x\geq 1`

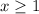
• The second inequality is given by:

`2x-7<5`

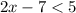
On adding both side of the inequality by 7 we get:

`2x<5+7\\\\i.e.\\\\2x<12`

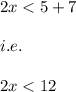
on dividing both side of the inequality by 2 we get:

`x<6`

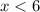
Hence, the solution of the compound inequality is:

`1\leq x<6`