Question

Which statements are true about the ordered pair (10, 5) and the system of equations?

(Look at the file to answer the question)

Answer 1

Answers:

The first and third options should be selected.

• The ordered pair (10,5) is a solution to the first equation because it makes the first equation true.
• The ordered pair (10,5) is not a solution to the system because it makes at least one of the equations false.

Step-by-step explanation:

In order for a point to be a solution to a system of equations, it must make both equations true when its x and y values are substituted in. That being said, we need to test if (10,5) is a solution for the two equations.

Let's try the first equation. Substitute 10 for x and 5 for y and solve:

`2x - 5y = -5\\2(10) - 5(5) = -5\\20 - 25 = -5\\-5 = -5`

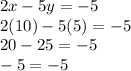
-5 does equal -5, so (10,5) is a solution to the first equation.

Next, let's test the second equation. Do the same:

`x + 2y = 11\\(10) + 2 (5) = 11\\10 + 10 = 11\\20 = 11`

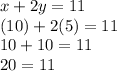
However, 20 does not equal 11, therefore (10,5) is not a solution to the second equation.

So far, we know that (10,5) is a solution to the first equation, but not the second equation. Knowing this, it cannot be a solution to the system because it does not make both equations true. Therefore, only the first and third options should be selected.