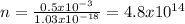Answer:
The number of photons is 4.8x10^14
Step-by-step explanation:
The frequency of wave is equal to:
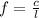
where c is the speed of light, l is the wavelength of wave. Replacing values:
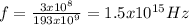
The energy of the proton is:
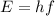
where h is the Planck´s constant. Replacing
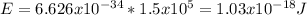
The number of photons is:
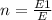
where E1 is the energy of photon. Replacing: