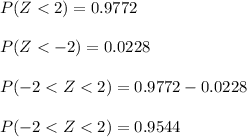Question

At an intersection, the red light times are normally distributed with a mean time of 3 minutes and a standard deviation of 0.25 minutes. Approximately what percent of red lights last between 2.5 and 3.5 minutes?

Answer 1

P = 95%

Explanation:

The average is:

`\mu = 3\ minutes`

The standard deviation is:

`\sigma = 0.25\ minutes`.

We want the probability that the red light lasts between 2.5 minutes and 3.5 minutes

This is:

`P(2.5 <X <3.5)`

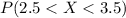
Now we must transform these values to those of a standard normal distribution to facilitate calculation by using the probability tables.

`P(2.5-3 <X- \mu<3.5-3)\\\\P((2.5-3)/(0.25) <(X- \mu)/(\sigma)<(3.5-3)/(0.25))\\\\P(-2<Z<2)`

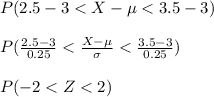
This is:

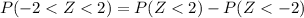`P(-2 <Z <2) = P(Z <2) - P(Z <-2)` ---------- (By the symmetry of the standard normal distribution)

When you search for the normal standard table, you get the following value:

`P(Z <2) = 0.9772\\\\P(Z <-2) = 0.0228\\\\P(-2 <Z <2) = 0.9772 - 0.0228\\\\P(-2 <Z <2) = 0.9544`