Question

A power line extends from a light pole 43 meters to the ground and makes an angle of 60 degrees with the ground. To the nearest tenth of a meter, how tall is the light pole?

Answer 1

Answer: 37.2 meters

Explanation:

The triangle shown in the image attached is a right triangle.

Therefore, to calculate the height of the light pole (x), you can apply the proccedure shown below:

-Apply
`sin\alpha=(opposite)/(hypotenuse)`

-Substitute values.

-Solve for the height of the light pole (x).

Then you obtain the following result:

`sin\alpha=(opposite)/(hypotenuse)\\\\sin(60\°)=(x)/(43)\\\\x=43*sin(60)\\x=37.2`

Answer 2

37.2 meters

Explanation:

Since this is the right triangle, the side that is 43 m is the hypotenuse.

Note: the side opposite of 90 degree angle is hypotenuse.

Also, we want the height of the pole, which is the side that is "opposite" to the angle 60 degree given.

Now, which trigonometric ratio relates "opposite" and "hypotenuse"??

It is SINE. Now we can write and solve (let the height of the pole be h):

`Sin(\theta)=(Opposite)/(Hypotenuse)\\Sin(60)=(h)/(43)\\h=Sin(60)*43\\h = 37.24`

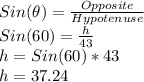
The light pole is 37.24 meters tall, to the nearest tenth, it is 37.2 meters