Question

Farimah and Helio are standing 15 ft. apart from each other and looking up at a kite that is with the flying between them. Farimah is flying the kite on a 57 ft. string at an angle of 68° with the ground. How far is Helio from the kite?

A. 64.1 ft.
B. 56.2 ft
C. 60.0 ft.
D. 53.2 ft.

Answer 1

D. 53.2

You can use the Law of Cosines to solve.

Answer 2

D. 53.2 ft.

Explanation:

As you can see in the diagram, Farimah, Helio, and the kite are making a triangle. We know from our problem that the distance from Farimah to Helio is 15 ft, the distance from Farimah to the kite is 57 ft, and the angle of elevation from Farimah to the kite is 68°. From this situation, we can infer that we have two sides of the triangle and the angle between those sides; therefore, we can use the law of cosines to find the third side, which is the distance form Helio to the kite:

`c^2=a^2+b^2-2abcos(C)`

`c^2=57^2+15^2-2(57)(15)cos(68)`

`c=√(57^2+15^2-2(57)(15)cos(68))`

`c=53.2`

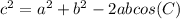
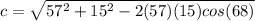
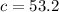
We can conclude that Helio is 53.2 ft from the kite.