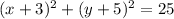Answer:
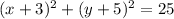
Explanation:
The equation of the in center-radius form is the shown below:

Where the center is at the point (h,k) and r is the radius of the circle.
Based on the information given in the problem, the center of the circle is at the point (-3,-5) and a radius of the circle is 5, then, you must substitute values into the equation shown above.
Therefore, you obtain the following equation: