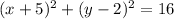Answer: OPTION A
Explanation:
Apply the formula for calculate the distance between two points to know the value of the diameter of the circle:
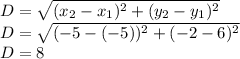
The equation of the circle is standard form is:

Where r is the radius and (h,k) is the point of the center of the circle.
As we know the diameter, we can find the radius:
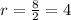
Substitute it into the equation:
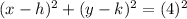
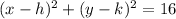
Then, the answer is: