2 Answers

Mythio · Answer 1 · 2020-12-10T19:29:32+0000

Answer:

(A)
$x=60^(\circ)$

Explanation:

Given: It is given that the circle P is circumscribed about quadrilateral ABCD and ∠BCD=120°.

To find: The value of x

Solution: It is given that the circle P is circumscribed about quadrilateral ABCD and ∠BCD=120°.

Now, we know that the sum of opposite angles in a cyclic quadrilateral is 180°, therefore

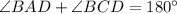
${\angle}BAD+{\angle}BCD=180^(\circ)$

substituting the given values, we get

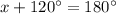
$x+120^(\circ)=180^(\circ)$

$x=60^(\circ)$

Thus, the value of x is 60°.

Hence, option A is correct.

Please log in or register to add a comment.