QUESTION 1
The tangent ratio is the ratio of the length of the opposite side to the length of the adjacent side.
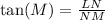
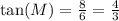
QUESTION 2.
We again use the tangent ratio to find angle S.
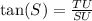
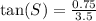
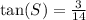
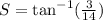

to the nearest hundredth.
QUESTION 3
We can find CE using the tangent ratio.
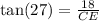
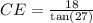
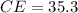
to the nearest 0.1.