Question

A toy robot is lauched at an intiial velocity of 80 ft/sec at an angle of 80 degrees with the horizontal. How long will it take the rocket to be 10 feet horizontally from its starting point? What will its vertical distance be at that point?

Answer 1

The robot has acceleration vector

`\vec a=-g\,\vec\jmath`

where
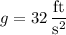`g=32\,(\rm ft)/(\mathrm s^2)` is the acceleration due to gravity.

Its initial velocity is

`\vec v_0=\left(80(\rm ft)/(\rm s)\right)\cos80^\circ\,\vec\imath+\left(80(\rm ft)/(\rm s)\right)\sin80^\circ\,\vec\jmath`

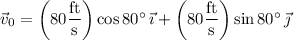
so that its velocity at time
`t` is

`\vec v=\vec v_0+\displaystyle\int_0^t\vec a\,\mathrm du`

`\vec v=\left(\left(80(\rm ft)/(\rm s)\right)\cos80^\circ\,\vec\imath+\left(80(\rm ft)/(\rm s)\right)\sin80^\circ\,\vec\jmath\right)+\left(-gt\,\vec\jmath\right)`

`\vec v=\left(80(\rm ft)/(\rm s)\right)\cos80^\circ\,\vec\imath+\left(\left(80(\rm ft)/(\rm s)\right)\sin80^\circ-gt\right)\,\vec\jmath`

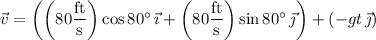
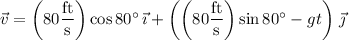
If we take the robot's starting position to be the origin, so that
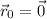`\vec r_0=\vec 0`, then its position vector at time
`t` is

`\vec r=\vec0+\displaystyle\int_0^t\vec v\,\mathrm du`

`\vec r=\left(80(\rm ft)/(\rm s)\right)\cos80^\circ\,t\,\vec\imath+\left(\left(80(\rm ft)/(\rm s)\right)\sin80^\circ\,t-\frac g2t^2\right)\,\vec\jmath`

The rocket is 10 feet horizontally away from its starting point when

`\left(80(\rm ft)/(\rm s)\right)\cos80^\circ\,t=10\implies t=0.72\,\mathrm s`

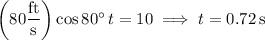
At this point, its vertical position is

`\left(80(\rm ft)/(\rm s)\right)\sin80^\circ(0.72\,\mathrm s)-\frac g2(0.72\,\mathrm s)^2=48\,\mathrm{ft}`

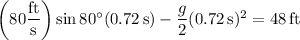
above the ground.