Question

asked May 7, 2020 154k views

2 Answers

Rick Donnelly · Answer 1 · 2020-05-11T03:20:19+0000

Answer:

The rectangular prism has the greatest surface area

Explanation:

Verify the surface area of each container

case A) A cone

The surface area of a cone is equal to

$SA=\pi r^(2) +\pi rl$

we have

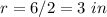$r=6/2=3\ in$ ----> the radius is half the diameter

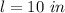
$l=10\ in$

substitute the values

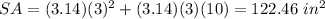
$SA=(3.14)(3)^(2) +(3.14)(3)(10)=122.46\ in^(2)$

case B) A cylinder

The surface area of a cylinder is equal to

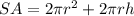
$SA=2\pi r^(2) +2\pi rh$

we have

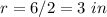$r=6/2=3\ in$ ----> the radius is half the diameter

$h=10\ in$

substitute the values

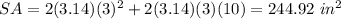
$SA=2(3.14)(3)^(2) +2(3.14)(3)(10)=244.92\ in^(2)$

case C) A square pyramid

The surface area of a square pyramid is equal to

SA=b^(2) +4[(1)/(2)bh]

we have

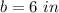$b=6\ in$ ----> the length side of the square

$h=10\ in$ ----> the height of the triangular face

substitute the values

$SA=6^(2) +4[(1)/(2)(6)(10)]=156\ in^(2)$

case D) A rectangular prism

The surface area of a rectangular prism is equal to

SA=2b^(2) +4[bh]

we have

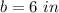$b=6\ in$ ----> the length side of the square base

$h=10\ in$ ----> the height of the rectangular face

substitute the values

$SA=2(6)^(2) +4[(6)(10)]=312\ in^(2)$

Please log in or register to add a comment.