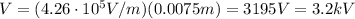(a)
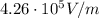
In a velocity selector, the speed of the beam is related to the magnitude of the electric field and of the magnetic field by the formula:
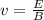
where
E is the magnitude of the electric field
B is the magnitude of the magnetic field
In this problem, we have
 (magnetic field)
(magnetic field)
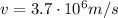 (speed of the particles)
(speed of the particles)
Solving the equation for E, we find the electric field:
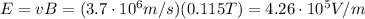
(b) 3.2 kV
The relationship between electric field and potential difference between the two plates is:

where, in this problem:
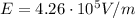 is the magnitude of the electric field
is the magnitude of the electric field
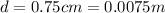 is the separation between the plates
is the separation between the plates
Substituting into the equation, we find the potential difference: