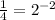Answer:
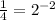
Explanation:
We are given the following expression and we are to write an equivalent exponential equation for it:
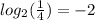
We know that the standard form of a logarithmic expression is given by:
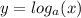
which is equivalent to the exponential form
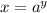 .
.
Therefore, for the given expression, the equivalent exponential equation will be: