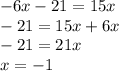Answer:
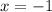
Explanation:
By the negative exponent rule, you have that:

By the exponents properties, you know that:
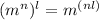
Therefore, you can rewrite the left side of the equation has following:

Descompose 32 and 8 into its prime factors:
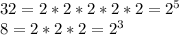
Rewrite:
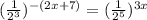
Then:
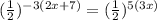
As the base are equal, then:
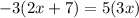
Solve for x: