Answer: Third option is correct.
Explanation:
Since we have given that
ABC is triangle with its dimensions:
AB = 11
AC = 13
∠A = 108°
BC = a
We need to find the length of 'a'.
So, we can use "Law of cosines" as we have given two sides and one angle.
So, it becomes,
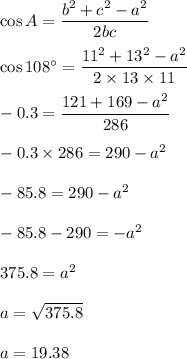
Hence, Third option is correct.