Question

What is the solution to the system of equations? 3x-6 = -12

x-2y = -8

(a) Use the substitution method to justify that the given system of equations has no solution.
(b) What do you know about the two lines in this system of equations?

Answer 1

Part a) In the procedure

Part b) Line A and Line B are different parallel lines

Explanation:

Part a) we have

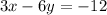`3x-6y=-12` ----> equation A

`x-2y=-8` ----> equation B

Isolate the variable x in the equation B

`x=2y-8`

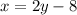
Substitute the value of x in the equation A

`3(2y-8)-6y=-12`

`6y-24-6y=-12`

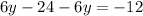
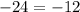`-24=-12` ------> is not true

therefore

The system of equations has no solutions

Part b) What do you know about the two lines in this system of equations?

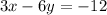`3x-6y=-12` ------> equation A

isolate the variable y

`6y=3x+12`

`y=(1/2)x+2`

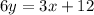
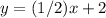
`x-2y=-8` -------> equation B

isolate the variable y

`2y=x+8`

`y=(1/2)x+4`

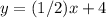
Line A and Line B are parallel lines, because their slopes are the same

Line A and Line B are different lines because their y-intercept is not the same