Question

A container is in the shape of a rectangular prism with a square base. It has a volume of 99 cubic inches and a height of 11 inches. How many softballs with a diameter of 3.8 inches will fit into the container? Use the drop-down menus to explain your answer.

Answer 1

Zero softballs with a diameter of 3.8 inches will fit into the container as length of the container is less the diameter of the softball.

Zero softballs can fit in length and zero softballs will fit in width.

Explanation:

Length of the square base in rectangular pyramid = s

Breadth of the square base in rectangular pyramid = s

Height of the square base in rectangular pyramid ,l = 11 inches

Volume of the square base in rectangular pyramid ,V=
`99 inches^3`

Volume of the cuboid = l × b × w

V= s × s × l

`99 inches^3=s^2* 11 inches`

s = 3 inches

Softballs with a diameter of 3.8 inches.

But the length of the container is less the diameter of the softball which means not even single ball will not be able to get into the container. So zero softballs can fit in length and zero softballs will fit in width.

Answer 2

A total of zero softballs will fit into the container

Explanation:

step 1

Find the dimensions of the base of the prism

we know that

The volume of the prism is equal to

`V=Bh`

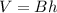
where

B is the area of the base

h is the height of the prism

In this problem we have

`V=99\ in^(3)`

`h=11\ in`

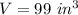
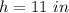
substitute in the formula and find the area of the base B

`99=B(11)`

`B=99/11=9\ in^(2)`

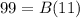
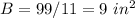
the length side of the square base is the square root of the area

so

`√(9)=3\ in`

we have that

The diameter of the softball 3.8 inches will fit (11/3.8=2.89 ) 2 times in the length of the container

The diameter of the softball 3.8 inches will fit 0 times in the width of the container

so

A total of 0 times of softballs will fit in the width of the container

therefore

A total of zero softballs will fit into the container