Question

Jason and Shawn both begin riding their bikes from different points away from the ranger station. They both ride their bikes in the direction away from the station along the same trail.

Jason starts biking 1 mile away from the station. Jason rides at a constant speed of 20 miles per hour.

Shawn starts biking 2 miles away from the station. Shawn rides at the same constant speed as Jason.

Let y represent the total miles away from the station.

At how many hours, x, will it take Shawn to catch up with Jason?

Based on the information provided, complete each expression below and determine the number of solutions.

Answer 1

No Solution

Explanation:

Jason starts riding a bike 1 mile from the station.

Shawn starts biking 2 miles from the station.

For Jason to reach Shawn, he needs to go at a higher speed. However we know that they are going at the same constant speed. Then they will never meet.

To prove it we propose the following system of equations. Where x is the hours and y is the distance in miles.

For Jason we have:

`y = 20x + 1`

For Shawn

`y = 20x + 2`.

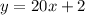We want to know when Jason and Shawn are at the same distance.

Then we write the following eqution and clear x:

`20x + 1 = 20x + 2\\20x -20x + 1 = 2\\1 = 2`

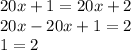
Equality is not satisfied. Therefore, it has no solution.

I mean Jason and Shawn will never be the same distance