Question

For each hour he babysits, Anderson earns $1 more than half of Carey’s hourly rate. Anderson earns $6 per hour. Which equation can be used to solve for Carey’s hourly rate, c?

Answer 1

10$ an hour

Explanation:

1/2c=6-1

c=2(6-1)

c=10

Answer 2

Carey's hourly rate to babysit is $10.

Explanation:

Given is -

For each hour he babysits, Anderson earns $1 more than half of Carey’s hourly rate.

Let Carey's hourly rate to babysit be represented by 'c'

So, Anderson's hourly rate will be =
`(c)/(2)+1`

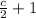
Also given that Anderson earns $6 per hour.

So, equaling both, we get;

`6=(c)/(2)+1`

`6=(c+2)/(2)`

`c+2=12`

`c=10`

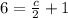
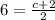
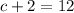
Hence, Carey's hourly rate to babysit is $10.