- Magnitude: 12.1 N.
- Direction: 17.0° to the 8 N force.
Step-by-step explanation
Refer to the diagram attached (created with GeoGebra). Consider the 5 N force in two directions: parallel to the 8 N force and normal to the 8 N force.
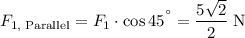 .
.
 .
.
The sum of forces on each direction will be the resultant force on that direction:
- Resultant force parallel to the 8 N force:
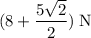 .
. - Resultant force normal to the 8 N force:
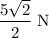 .
.
Apply the Pythagorean Theorem to find the magnitude of the resultant force.
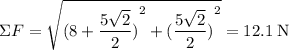 (3 sig. fig.).
(3 sig. fig.).
The size of the angle between the resultant force and the 8 N force can be found from the tangent value of the angle. Tangent of the angle:
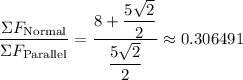 .
.
Find the size of the angle using inverse tangent:
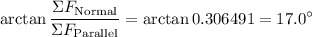 .
.
In other words, the resultant force is 17.0° relative to the 8 N force.