Answer:
3 m^2
Explanation:
The shaded area = Area of Sector - Area of Triangle
First we find area of sector =
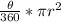
We know the angle is 60° (vertical angles are equal) and the radius is 6, so we find the area of sector to be:
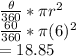
Secondly, we find area of triangle (isosceles) =
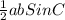
where
a and b are the two sides bordering the known angle, so a=b=6, and
C is the angle which is 60°
Now we find the area to be:

Hence, area of shaded =
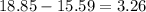
Rounding to nearest whole number, area of shaded region = 3m^2