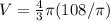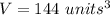Question

The volume of a cylinder cone and sphere are shown below. The three figures have the same radius. The cylinder and the cone have the same height with h = r.

if the volume of the cone is 36 cubic units, what are the volumes of the cylinder and sphere? Explain your answer.

answers: Cylinder:_______cubic units
sphere:________cubic units
________________________________________________________________________________________________________________________________________________________________________________________________________________________________________________________________________________________________________________________________

Answer 1

Part 1) The volume of the cylinder is
`V=108\ units^(3)`

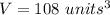
part 2) The volume of the sphere is
`V=144\ units^(3)`

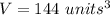
Explanation:

step 1

Find the radius of the cone

we know that

the volume of the cone is equal to

`V=(1)/(3)\pi r^(2) h`

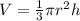
we have

`V=36\ units^(3)`

`h=r\ units`

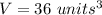
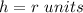
substitute and solve for r

`36=(1)/(3)\pi r^(2) (r)`

`108=\pi r^(3)`

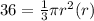
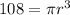
`r^(3)=108/ \pi` ------> equation A

step 2

Find the volume of the cylinder

we know that

the volume of the cylinder is equal to

`V=\pi r^(2) h`

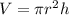
we have

`h=r\ units`

substitute

`V=\pi r^(2) (r)`

`V=\pi r^(3)\ units^(3)`

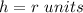
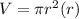
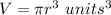
substitute the equation A in the formula above

`r^(3)=108/ \pi` ----> equation A

`V=\pi (108/ \pi)\ units^(3)`

`V=108\ units^(3)`

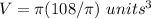
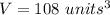
step 3

Find the volume of the sphere

we know that

The volume of the sphere is equal to

`V=(4)/(3)\pi r^(3)\ units^(3)`

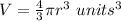
substitute the equation A in the formula above

`r^(3)=108/ \pi` ----> equation A

`V=(4)/(3)\pi (108/ \pi)`

`V=144\ units^(3)`