Answer:
The correct option is 3.
Explanation:
The parent function is
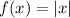
The horizontal stretch and compression is defined as
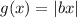
If 0<b<1, then it is horizontal stretch and if b>1, then it is horizontal compression.
Graph 1 passing thought the point (1,-1).

This statement is false for any value of b, because the value of modulus can not be negative.
Graph 2 passing thought the point (1,2).

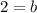
Since b>1, therefore this graph represent the horizontal compression.
Graph 3 passing thought the point (2,1).
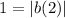
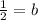
Since 0<b<1, therefore this graph represent the horizontal stretch.
Thus, the correct option is 3.