Question

Find the sum of the first 100

Answer 1

The terms of an arithmetic sequence are generated by adding a fixed term
`r` every time.

So, we start with
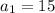`a_1=15`, and we continue with
`a_2=15+r`,
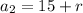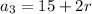`a_3=15+2r` and so on.

As you can see, the general rule is
`a_n = 15+(n-1)r`

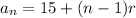
With this information, we can derive
`r`, knowing that

`a_(100) = 307 = 15+99r \iff 99r = 292 \iff r = (292)/(99)`

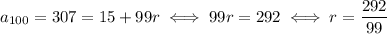
So, the sum of the first 100 terms is

`[tex]\displaystyle \sum_(i=0)^(99) 15+i(292)/(99) = \displaystyle \sum_(i=0)^(99) 15 + \displaystyle (292)/(99)\sum_(i=0)^(99) i = (15\cdot 99) + (292)/(99)(99\cdot 100)/(2) = 1485 + (490342)/(99)`