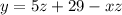Answer:

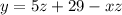
Explanation:
Given:
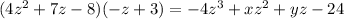 ; find x and y.
; find x and y.
Let's start with finding x first. Let's distribute the left side of the equation.
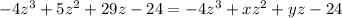
Let's move everything we can to the left side of the equation. Some things will cancel out.

Now, let's move the term that includes
 to the left side of the equation. Everything else should be moved to the right.
to the left side of the equation. Everything else should be moved to the right.
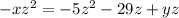
Let's get rid of the negative sign in front of
 by dividing both sides by -1.
by dividing both sides by -1.
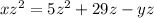
To get
 completely isolated, divide both sides by
completely isolated, divide both sides by
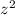 .
.
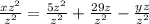
Simplify.

Let's solve for y. We will start with the distributed and simplified equation to make it easier.

Now, let's move the term that includes
 to the left side of the equation. Everything else should be moved to the right.
to the left side of the equation. Everything else should be moved to the right.

Again, let's get rid of the negative sign in front of the
 by dividing both sides by -1.
by dividing both sides by -1.
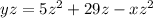
To isolate y, divide both sides by z.
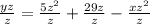
Simplify.