Step-by-step explanation:
Kepler has given its three laws. The third law of Kepler gives the relationship between the orbital period and the distance between the satellite and the center of orbit. Mathematically, it is given by :
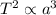
or
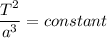
It is clear from above formula, that as the distance between a satellite in a circular orbit and the central object increases, the period of the satellite also increases.