Answer:
Option A) 4.4 is the correct standard deviation of the the given data set.
Explanation:
We are given the following data set:
127, 135, 128, 131, 133, 127, 122
Formula:
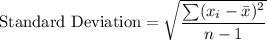
where
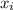 are data points,
are data points,
 is the mean and n is the number of observations.
is the mean and n is the number of observations.
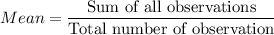
Solving:

Sum of squares of differences = 4 + 36 + 1 + 4 + 16 + 4 + 49 = 114
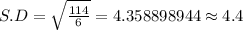
Option A) is the correct standard deviation of the the given data set.