Answer:
The correct choice is B.
Explanation:
a. Given

We can subtract
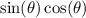 from both sides to obtain;
from both sides to obtain;

We can then factor
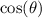 to obtain;
to obtain;
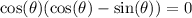
We can then proceed with our solution using the zero product principle.
b. Given
 , it is not valid to divide both sides by
, it is not valid to divide both sides by
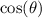 because
because
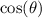 could be equal to zero and division by zero in disguise will not result in the true solutions of the given equation.
could be equal to zero and division by zero in disguise will not result in the true solutions of the given equation.
c. To solve sin2θ+2cosθsin2θ=0, first factor out the common factor of sin2θ on the left side.
This is also a justifiable approach.
d. To solve the equation sinθ+cosθ=1, first square both sides of the equation.
Squaring both sides will help solve the equation with double angle properties easily.